Further Mechanics Collisions part 2
Hi,
Today I'll talk about how I plan to use two more Geogebra pages to teach collisions between two particles.
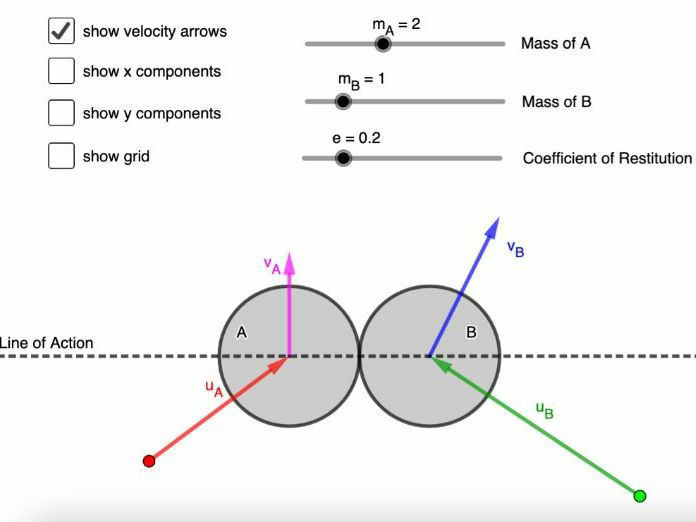
I'll call them A and B:
In my previous post I talked about particle-barrier collisions and how I plan to introduce Newton's experimental law.
Before starting with two particles, I'm assuming students should be familiar with conservation of momentum and have seen Newton's experimental law.
Starter:
Conservation of momentum question - direct collision. No diagram given and deliberately confusing. I want students to draw a diagram and solve the problem using what they already know from M1.
I'll invite fast finishers to think about how this connects to the e value from the previous lesson. Can they remember the formula?
Calculate e for this example:
Write formula up in general terms (ie in terms of approach speed and separation speed).
Then show the calculation and invite a weak student to suggest a general version in terms of velocities.
Draw and label a general diagram, with formula and students copy it down.
So direct two-particle collisions are done. Practice that.
Tell the class that next lesson we'll look at indirect collisions and set this homework where they play with tool "A":
1) A direct collision question, given initial velocities and the e value, find the final velocities. They have to draw a good diagram and show all their calculations.
2) they use the tool to check the answer, take a screenshot and compare it to their diagram
3) next change the question so that it is an indirect collision question, but the components parallel to the line of action are the same as the first question. Get students to look at the components of the speeds before and after collision and think about how to calculate them if you are only given initial velocities and the e value.
Start the next lesson going over the homework and hopefully the students will be ready to suggest a method for structuring solutions for these sorts of questions.
What you want them to remember is that parallel to the line of action, use components of velocity and treat it like a direct collision. Perpendicular to the line, there is no action, so nothing changes.
Don't forget to stick the components together again when you're done
Oblique collision example 1 on the board, sign-posting the method. Make sure students are copying the instructions as well as the calculations.
Example 2 is very similar but one particle has different mass. Tell the class to try it, and leave example one written on the board. Then after some time, you can use a different pen to amend the first example or ask a volunteer to do it.
Students try some controlled practice questions. Homework is more practice. Check the homework carefully and use more classtime for this topic if necessary.
Tool "B" allows you to animate the collision and it has a hidden billiards table. Some suggestions might be:
- hide/show the line of action and ask students why ball B only moves along the line of action- set up a direct collision and ask students to predict what will be different for different values of e.- same with an indirect collision- challenge a student to a game of billiards (you can use the LoA for your shot and hide it on his turn, for a laugh). Move the balls into different positions for direct and indirect collisions, and ask students lots of "what if" questions about different e values and where the white ball ends up.- ask students how realistic the model is. Show videos of snooker trick shots then try to reconstruct them on the tool. Real snooker players can put spin on the ball, so real life is more complicated. Could we improve the model? How? Lots to discuss here...
If you have better ideas please get in touch. I hope some of this is useful.

Comments
Post a Comment